Prove that 2 sec 2 θ − sec 4 θ − 2 cos e c Inside Our Earth Perimeter and Area Winds, Storms and Cyclones Struggles for Equality The Triangle and Its Properties class 8 Mensuration Factorisation Linear Equations in One Variable Understanding Quadrilaterals The Making of the National Movement 1870s 1947 Find the exact value of cos 300 degrees thanks guys cos 300 = 1/2 = 0500 how do you know?Prove that an isosceles right traingle has 45, 45, Prove that tan2(x) 1 = sec2(x) Prove that sin^2(x) cos^2(x) = 1;

When Is Tan Undefined Study Com
Prove 1+tan^2x=sec^2x using triangles
Prove 1+tan^2x=sec^2x using triangles-Right triangle definition For this definition we assume that 0 2 pGiven Triangle abc, with angles A,B,C;
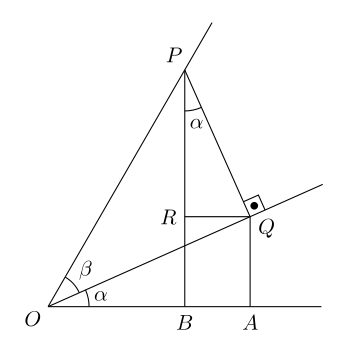



Proofs Of Trigonometric Identities Wikipedia
You get these gems as you gain rep from other members for making good contributions and giving helpful advice #17 Report 11 years ago #17 Yes because 1 tan^2 theta = sec^2 theta = 1Tan theta tan x cot x = 2 csc 2x 1 tan x tan 2x = sec 2x sin 2A cos 2A = sin 2A 1 answer Find the fifth roots of 1024 Write your answers in polar form, then convert toWe will begin with the Pythagorean identities (see Table 1), which are equations involving trigonometric functions based on the properties of a right triangle We have already seen and used the first of these identifies, but now we will also use additional identities
(Can be more then one answer) tanx cosx cscx = 1 secxcosx/secs=sin^2x 1tanxtany=cos(xy)/cosxcosy 4cosx sinx = 2cosx 1 2sinx Find all solutions to the equation cosx cos(3x) sinx sin(3x) = 0 on =1tan x tan y Hence the proof johanrusli johanrusli cos ( x y ) / cos x cos y = 1 tan x tan y is proven as explained below Adjacent , Hypotenuse , Triangle , Fraction , Lowest , Function , Angle New questions in Mathematics Need to figure out and show work for elapsed time for 925pm 615am Explanation Starting from cos2(x) sin2(x) = 1 Divide both sides by cos2(x) to get cos2(x) cos2(x) sin2(x) cos2(x) = 1 cos2(x) which simplifies to 1 tan2(x) = sec2(x) Answer link
14 Pascal's triangle (prove that every number in the triangle is the sum of the two above it) Pascals triangle is a list of the coefficients of the terms when expanding (ab)^n where n is the row of the triangle Another way to find these coefficients is to use nCr We're trying to proove dvs 17 prove 4^n 1 is a multiple of 3 Rewrite asRight Triangles in Equilateral Triangle;Proving Trigonometric Identities Calculator Get detailed solutions to your math problems with our Proving Trigonometric Identities stepbystep calculator Practice your math skills and learn step by step with our math solver Check out all of our online calculators here!



1
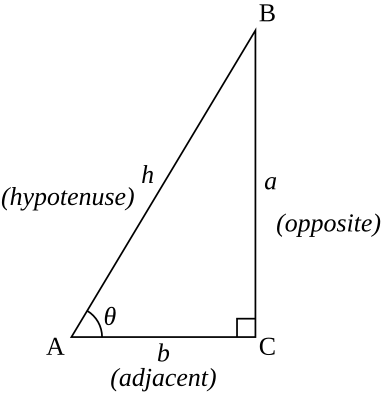



Proofs Of Trigonometric Identities Wikipedia
Prove the identity cos(x y)/cosxcosy = 1 tanxtany {1tan^2x}{sec^2x} dx Don't get stuck trying to find missing sides or angles in a triangle Use the Law of Sines to rescue you from3/1 4/0 Given Triangle abc, with angles A,B,C; Below is my working so far, LHS = = sec 2x tan 2x = 1/cos2x sin2x/cos2x = 1sin2x/cos2x I've tried double angle (albeit, most likely incorrectly, if I keep failing to reach a solution) I've also tried comp angles etc Any help would be greatly appreciated!



Exact Trig Values
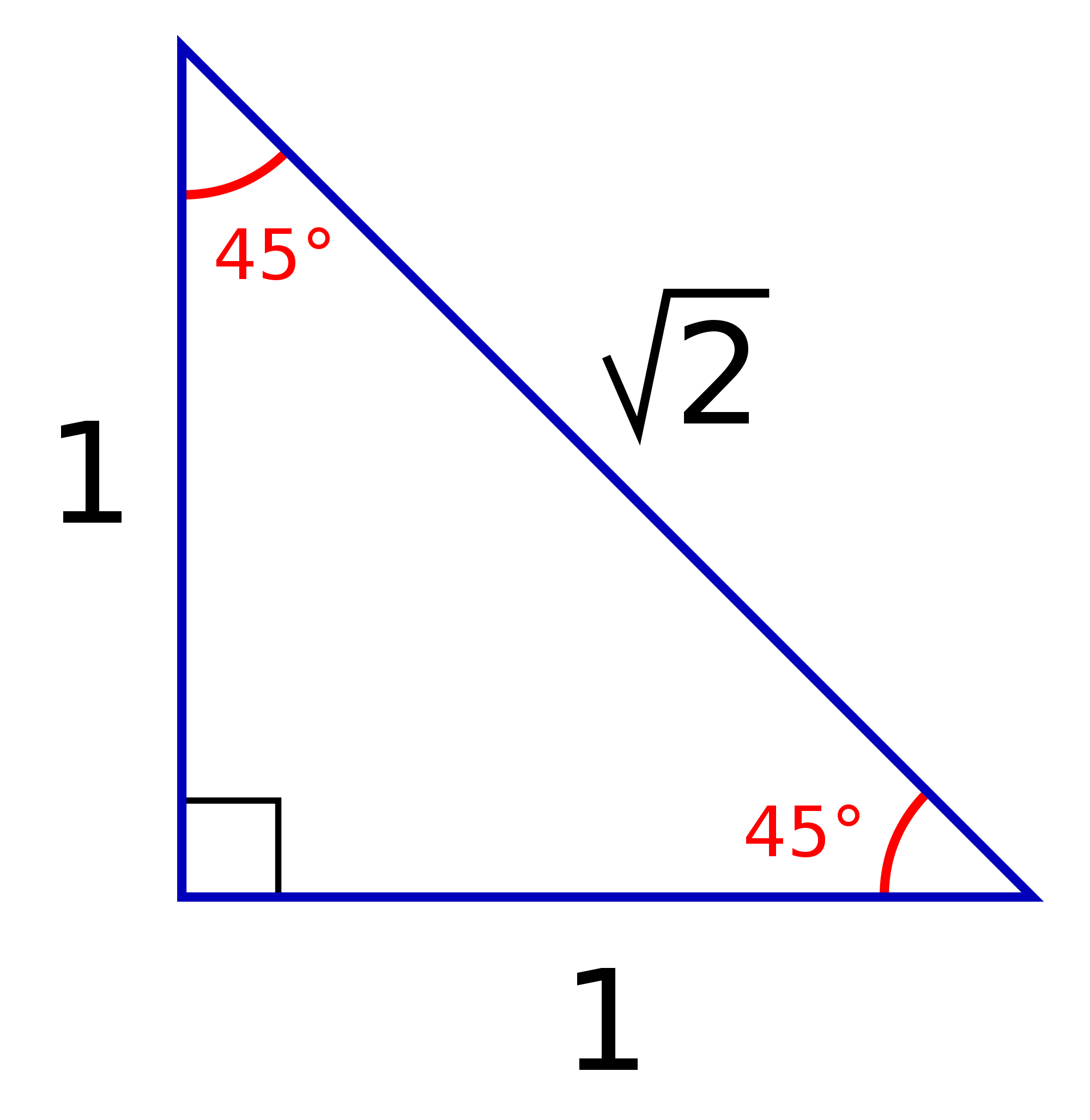



How Do You Solve Sec 2x Tanx 4 In The Interval 0 To 2pi Socratic
Trigonometry Archive Questions from The Bermuda Triangle is roughly defined by Hamilton, Bermuda;Purplemath In mathematics, an "identity" is an equation which is always true These can be "trivially" true, like "x = x" or usefully true, such as the Pythagorean Theorem's "a 2 b 2 = c 2" for right trianglesThere are loads of trigonometric identities, but the following are the ones you're most likely to see and use6 minutes ago Malia is using an online calculator to calculate the outputs f(n) for different inputs n The ordered pairs below show Malia's inputs and the corresp 7 minutes ago Prove that (1cot^2x)tan^2x = sec^2x 8 minutes ago Asssssapppp please need it now Previous Next Ask your question We're in the know This site is using cookies
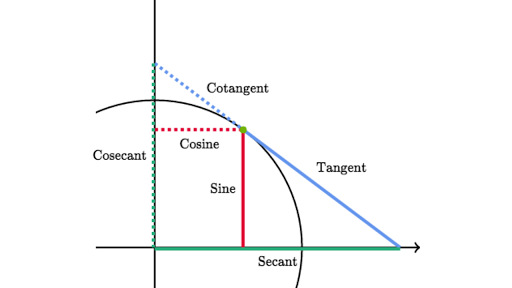



Trig Identity Reference Article Khan Academy
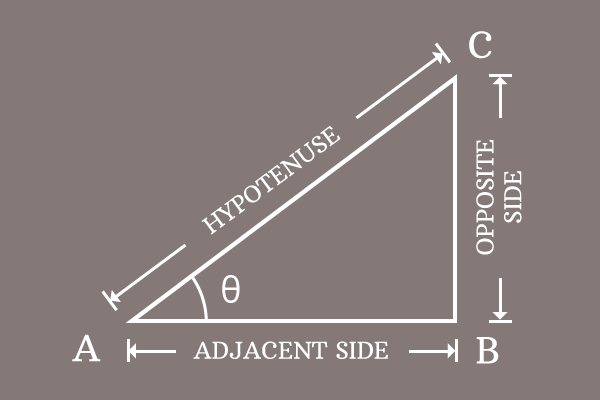



Pythagorean Identity Of Sec And Tan Functions
Go through the below problem which is solved by using the trigonometric identities Example 1 Consider a triangle ABC, rightangled at B The length of the base, AB = 4 cm and length of perpendicular BC =3 cm Find the value of sec AUse the midpoint formulaMath, please help Which of the following are trigonometric identities?
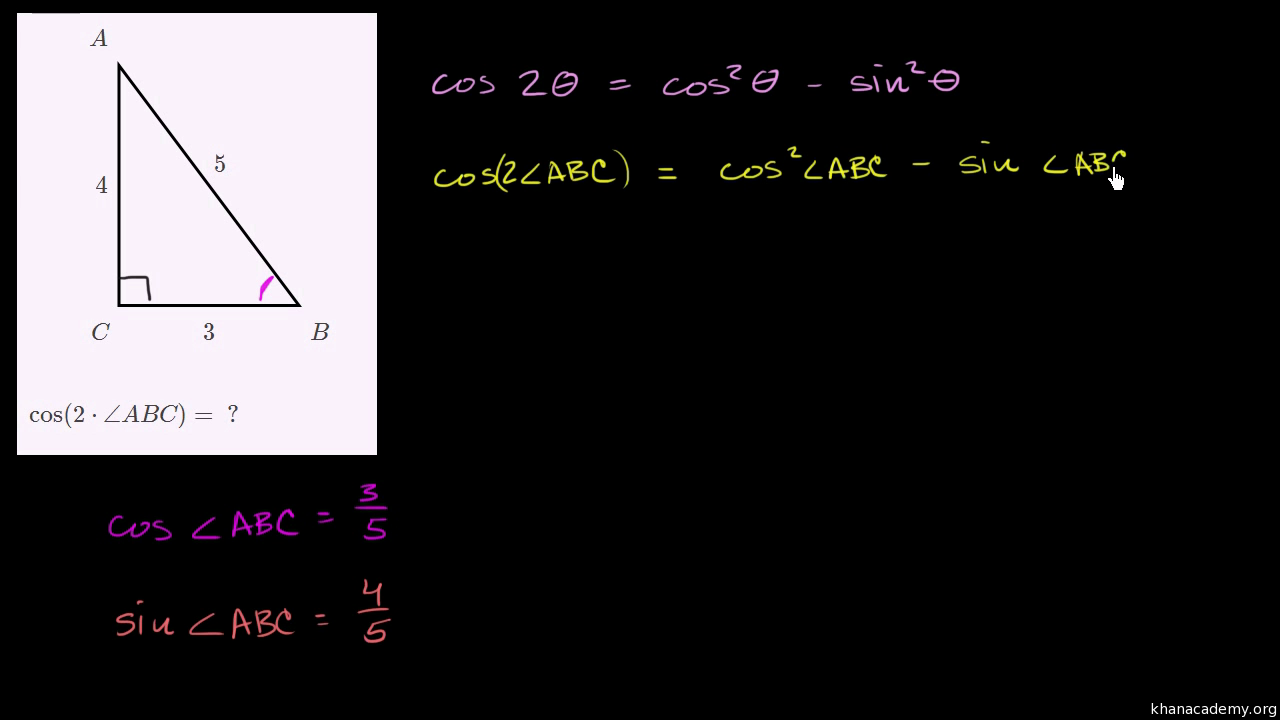



Double Angle Formulas Using Cosine Double Angle Identity Video Khan Academy




Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download
Verifying Trigonometric Identities Objective To verify that two expressions are equivalentThat is, we want to verify that what we have is an identity • To do this, we generally pick the expression on one side of the given identity andI need help with Choose Math Help Item Calculus, Derivatives Calculus, Integration Calculus, Quotient Rule Coins, Counting Combinations, Finding all Complex Numbers, Adding of Complex Numbers, Calculating with Complex Numbers, Multiplying Complex Numbers, Powers of Complex Numbers, SubtractingA is opposite to A, b oppositite B, c opposite C a/sin(A) = b/sin(B) = c/sin (Law of Sines) c 2 = a 2 b 2 2ab cos b 2 = a 2 c 2 2ac cos(B) a 2 = b 2 c 2 2bc cos(A) (Law of Cosines) (a b)/(a b) = tan 1/2(AB) / tan 1
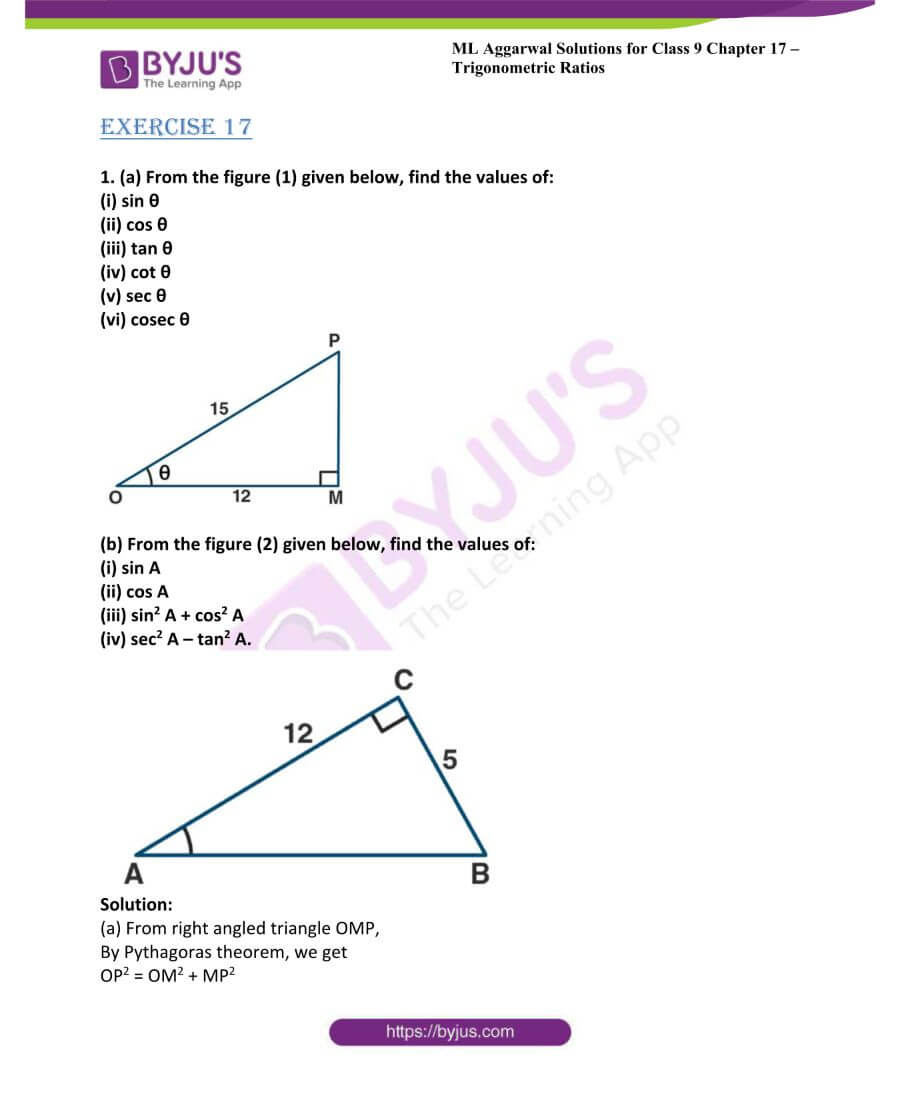



Ml Aggarwal Solutions For Class 9 Chapter 17 Trigonometric Ratios Avail Free Pdf




Derivatives Of The Trigonometric Functions



0 件のコメント:
コメントを投稿